The Day I Almost Won the Nobel Prize

I wrote the other day about how shocked I was to learn that the leading proponents of the “semi-classical school” (which by my reckoning should actually be called the hemi-semi-classical school) had such poor physical intuitions. If they knew what I knew about things like the crystal radio, they could never come up with such misguided ideas as using the size of an atom to estimate the capture cross-section of the photo-electric effect.

Actually, they shouldn’t even need to understand the crystal radio for that. When you study inelastic collisions in Grade Eleven, it’s perfectly obvious that the greatest momentum transfer occurs between two particles of comparable mass. When one is very much lighter than the other (as is the case for a “photon” colliding with an electron) there is very little energy transferred to the larger particle. The absorption of light is clearly a bulk phenomenon that is not concentrated on a single atom, so the atomic cross-section has no place in any such calculation.

Anyhow, when I read Scully’s misguided explanation of these things, it got me thinking again. It had been probably ten years since I first started going on the internet to argue the case for classical light in discussion groups like sci.physics. I’d challenge anyone who said that the photo-electric effect proved that light was made of particles. And whenever I got into a discussion, there were these two self-appointed guardians of the truth who always showed up to put me in my place. Either Jim Carr or Mati Meron would jump in and say something to the effect of “even if you can explain the photo-electric effect, you can’t explain the Compton effect”. It didn’t matter that I wasn’t trying to explain the Compton effect. The fact that I had no explanation for it made whatever I said about the photo-electric effect, in their eyes, irrelevant.

It did no good to challenge them to meet me head on and argue the photo-electric effect. It was always “even if you are correct…”, never an actual admission that I was in fact correct. I would never get anywhere until I explained how the Compton effect worked without photons.

And then it happened! I guess it was reading that article by Scully that got me riled up or something, but I was out walking in the forest one day when it came to me in a flash. The Compton effect was much easier to explain than the photo-electric effect! The mathematics are identical to one of the most familiar examples from first-year physics, the well-known case of the infinite square well.

Think about it: in the square well, you have standing waves of charge, which can be written as two travelling waves going in opposite directions. It means that if you have a single electron in the superposition of two states, one travelling to the left and one travelling to the right, that the electric charge distribution will consist of many parallel sheets.

You can see right away how such a charge distribution must interact with a classical electromagnetic wave. In general, there will be no net interaction. But in the special case when the wavelength of the light is equal to the wavelength of the momentum, something very interesting happens. There need only be the slightest reflection off any given sheet: the reflection from the second sheet will be perfectly in phase with the reflection from the first sheet, as will the reflections from all subsequent layers. In very short order the power of the incident light wave will be completely reflected. And that is just what you find in the Compton effect.

Of course, to get total reflection you have to analyze the experiment in a center-of-mass reference frame. In the ordinary lab reference frame you only get fractional reflection. But the rules are well known whereby you transform the calculation from one reference frame to the other, and the net result is total reflection in the center-of-mass frame. And the reason why it has to work is obvious: in quantum mechanics, wavelength is the the measure of momentum; and in the C-O-M frame, the wavelength of the light is equal to the wavelength of the electron. That’s when the interaction suddenly becomes ultra-strong.

I was ecstatic when I put all this together, and not only because I finally had an answer for my tormentors from the internet forums. This was much bigger! If I had actually found a classical explanation for the Compton Effect, this would surely overturn the whole paradigm upon which the Copenhagen Interpretation of quantum mechanics was built. Was my analysis correct? It was so obvious that I could hardly doubt it. Had anyone else come up with it before me? The idea was laughable. Surely if such an explanation had ever been proposed in the past, it would be widely known. On the contrary: in all the popular accounts, the Compton effect was hailed as the third “nail in the coffin” that laid to rest the classical theory of electromagnetism once and for all. (The other two “nails” being the black body spectrum and the photo-electric effect.)

My readers will undoubtedly be surprised and disappointed to learn that I was not, after all, awarded the Nobel Prize for this amazing discovery. The sad story of how this happened will be left for a future posting.

The Semi-Classical School of Jaynes and Scully

When I was in grad school back in the 90's and first started piecing together the picture of quantum atoms as tiny oscillating charge distributions, my professor told me that a fair amount of work had been done on that picture in the 1960's by someone named Jaynes. It seems that despite the simplifications prevelant in the popularizations, real physicists knew that things like the photo-electric effect could be explained on a phenomenological (descriptive) level just as well by the wave theory as by the particle theory of light. It seems that the Jaynes school set out to see just how far this could be carried through on a mathematical level as well.

So I understood that the basic ideas of my approach were already well-known in the physics community. It was only about two years ago that I learned how wrong I was.

It is pretty easy to set up a calculation in quantum mechanics using the wave theory of light. You have the same atoms, the same potential, the same Schroedinger equation; all you do add a new potential V=kz in the vicinity of the atom and allow the potential V to oscillate sinusoidally in time. If you can write the equation then in principle, the equation can be solved.

So far this is the same as my approach. What I would then go on to do is track the motion of the atomic charge in the presence of this oscillating field, see how the charge distribution also begins to oscillate, calculate the new field due to these oscillating charges according to Maxwell's equations, and add these new fields to the original "driving" field to get the resultant.

I learned only recently that this was not the method of the Jaynes school! What they did was simply to apply their oscillating field to an atom in the ground state, and then using the standard calculational techniques, determine the transition probabilites from the ground state to the excited state. They were still looking for the same old quantum leaps instead of following through on the actual time evolution of the process.

This was a pretty big disappointment. I had thought that the so-called "semi-classical approach" was what I and the Jaynes school had both been practising. It turns out that either they were really doing the "hemi-semi-classical approach", or I was actually doing the "75%-classical approach". Either way, we were not on the same page.

I learned this in the course of some very nasty arguments in physicsforums.com. I was originally trying to defend my picture of atomic antennas on its own merits, by logic. Finally I did what I hate to do and invoked authority to defend my ideas: "I'm only making the same argument that Jaynes made in the 1960's". I was shot down right away. There are no oscillating charge distributions in the Jaynes picture, only pure eigenstates. The jump from one pure eigenstate to another may be stimulated by an oscillating field instead of a "photon", but it still works on the basis of quantum leaps.

Was the Jaynes school then merely doing mathematical acrobatics, or did they have a physical picture like I did? My road to quantum mechanics began with the crystal radio. I had discovered a physical picture which showed me that energy is exchanged between two systems over a huge volume of space that has nothing to do with the physical dimension of the systems, but rather is based on the wave-on-wave interaction extending over dimensions measured in wavelengths. That is why a tiny crystal radio antenna can absorb quantities of power that are equivalent to an area of hundreds of square meters, even though the physical cross-section of the antenna may be measured only in square centimeters. Did the Jaynes school share these kinds of pictures with me? They did not!

I found this out conclusively about five years ago after reading the Wikipedia article on "photons". At the bottom of the article they linked to a video of the Nobel Prize lecture by Roy Glauber and several research papers on the "history of the photon", which I actually sent away for. The Glauber lecture was horrible, but that's another story. One of the articles in the package was by Scully, a leading member and perhaps the recognized heir to the Jaynes school, and I was shocked to read what he said about the photoelectric effect (and I'm paraphrasing here): that most of the phenomena associated with the effect can be equally well explained by the wave theory, but there is a problem with the absorption cross section! He then explicitly writes out the cross-sectional area of a typical atom and observes that the amount of radiant energy passing through this cross section is much too small to account for the rate of prompt emmision measured in typical experiments.

How does he not know that he is using the wrong cross section!? He obviously doesn't understand the crystal radio, or he would be using the wavelength of the light for his relevant cross-section, and he would get an area a million or so times greater. And this is just if he is talking about the photo-electric effect on a single atom, more precisely the photo-ionization effect. If he is talking about light striking a metal plate, he needs to use the whole area of the plate, because that is the size of the wave function of a single electron. It's appalling to me that people at the very top of the game in physics are working on such a sophisticated mathematical level without having the physical pictures to back it up.

Finally, I ought to mention that I don't like Scully. When my professor told me about the Jaynes school he said I might want to contact them about doing PhD research. I wrote a four-page handwritten letter to Scully outlining some of my ideas and waited eagerly for a response. Finally, after a few months I phoned him. He had no idea who I was. "Yes, I get a lot of letters but I don't really have time to read them". No apology. Maybe he doesn't have time, or maybe he read mine and thought I was a quack, but that's no excuse. Don't people like that have assistants to at least send out standard form rejection letters?

More on Perturbation Theory and Ladder Operators

One of the big surprises for me since I started following my blog statistics is the number of hits I've gotten for my musings on ladder operators. It's a topic I've never really understood properly, so when I started writing about it last April my thoughts were pretty speculative. Reading over it today however it seems to me I got off to a pretty good start and it might be worth going a little farther down that road. Hence today's article.

What really annoyed me in university was the day the prof said, right out of the blue, "let's define an operator Q such that Q operating on energy eignestate |n> returns state |n+1>". How does it do that? The professor couldn't care less. "We can define an operator to do anything we want". I have never understood what the hell that even means.

I can't talk about an operator unless I have some idea what it actually looks like. That's why in my last article I started off by trying to construct physical interpretations of the ladder operator. I thought I was doing a pretty good job, and in the end, I said that you take your hydrogen atom, put it between the plates of the capacitor, and the differential change when you add charge to the capacitor in effect creates a differential change in the ground state of the hydrogen atom; and that differential change is exactly proportional to the first excited state. So the operator which changes the ground state to the excited state is simply an electric field in the z direction.

Of course, no one who talks about ladder operators bothers to work them out for the hydrogen atom. They only care what they do to the harmonic oscillator. Interestingly enough, my definition works for the first transition of the harmonic oscillator, because when you multiply the wave function exp(-z^2) by z, you get essentially the first excited state. So in other words, so far so good.

The problem with this definition is that it doesn't exactly work on the next level. What operator takes the pz state and changes it to the d state? It seems that multiplication by z does not quite do the trick. It certainly doesn't do the trick for the harmonic oscillator, where you have to multiply by z and then add in some of the original ground state.

You can actually see that it can't work, because if it did the second excited state would be equal to the ground state multiplied by z^2. This can't be right because this new state isn't even orthogonal to the original ground state, as it must be if it is to be an eigenstate.

There's another very different way you can see that it doesn't work, and that is from the perspective of perturbation theory. It is true that perturbation theory lets us find the new ground state of an atom in a constant field by mixing in a bit of the pz state to the s state. But are all the new eigenstates found so easily? In other words, do we find the modified pz state simply by mixing in a bit of the d state? The answer turns out to be no.

The answer has to be yes for the perturbed s state because adding a bit of pz is the simplest possible perturbation you can make. And the energy change must be linear in the perturbation, because it is simply Hooke's Law applied to an atomic spring.

The perturbation of the second energy level looks a bit the same, because when you add a field in the z direction, you apply it to a charge distribution which is centered on the origin, so you must expect the charge distribution to be displaced along the z axis. The obvious way to do this is to mix in a bit of the d state; and this certainly works: but don't forget, you also get a displacement of the charge cloud when you add in some s state! In fact, it seems the true optimization is to mix in a bit of both the s and the d states. In fact, it's not even totally clear that the net energy of the perturbed state is greater or less than the unperturbed state. I'm thinking it's actually less.

Now I'm going to recall something I said in my April 6th post when I was fishing around for possible candidates for the ladder operator. I said that applying a field in the z direction was promising. When you apply this to an eigenstate, you basically multiply the state by z. But I also said that differentiating the state with respect to z was promising, because that was the first term in a Taylor expansion which basically displaces the state by a fixed amount.

Interestingly enough, when applied to the ground state, these two operations, differentiation and multiplication by x, have the same effect. Why not just add them together?

You can verify that this gives you the correct sequence of eigenstates for the harmonic oscillator. This patch turns out, it seems, to be just what is needed to fix the ladder operator altogether! It seems that when you apply both operations to higher levels, the effect of the second one is just to fix up the error left over by the first one. I'm not sure why or how it works, but it certainly seems to do the trick on the harmonic oscillator, and it just might work on the hydrogen atom as well.

It's all very confusing, and also quite speculative, but it seems to be a promising way to try and understand what is happening. I think I'm going to quit here and maybe six months from now I'll have some more to add.

A Visitor from Palestine

I mentioned last week that I had only just learned how Google Blogger provides amazing statistics concerning how many people have looked at your blog. Today I was delighted to note that I have had just this morning my first visitor from the Palestinian Territories. However, I am puzzled that my reports did not show another view for my article about the conflict, "Ten Things We Jews Believe about the Middle East". If you are still with us as I write these words, my friend, I urge you to check it out and please leave a comment.

How atoms are tiny antennas

In my last post I said the universe is full of tiny radio antennas, and they are called atoms. I'm sure people must wonder what I'm exactly talking about. There are few ideas in physics so strongly entrenched as the idea of the Bohr atom with its planetary orbitals and "quantum leaps": the principle that an electron can be in this orbital or that orbital, but nowhere inbetween; and that the mysterious leap from one orbital to the next is accompanied by the emission of a particle of energy called the "photon". How is this an antenna?

In 1926 the Bohr atom was replaced by the Schroedinger atom. The Bohr atom lasted all of eleven years but it is the iconic picture of an atom that everyone remembers. It is the symbol for atomic energy. But what about the Schroedinger atom? What does it look like? If you took high school chemistry, you might have seen pictures of cloudy-looking "orbitals: the s, the p, and the five "d" orbitals including the peculiar one with the equatorial ring. But the real beauty of the Schroedinger atom is what happens when you combine two orbitals at the same time. I've found a nice applet from the University of Saskatchewan that lets you see what they look like:

http://physics.usask.ca/~pywell/p381/qmatom/

If you clear the applet and then select the first s orbital and the first p orbital, you'll see the cloud of charge oscillating back and forth. That's what the charge is doing when the electron is in a superposition of two states. It looks just like an antenna, and everybody knows this. Schroedinger was ecstatic when he was able to show you didn't need the quantum leap to explain atomic transitions, and you didn't need to throw out Maxwell's Equations to explain radiation.

But incredibly, the other physicists scoffed at Schroedinger's interpretation! Heisenberg, Born, and Lorentz were firm believers in their own alternative interpretation based on probabilities and wave function collapse. This philosophy was an outgrowth of the original Bohr paradigm with its mysterious quantum leaps, concocted to accomodate the Schroedinger equation without allowing for the physical reality of the Schroedinger picture. I never understood how deeply ingrained these prejudices were until after being shot down in numerous internet forum arguments by physicists who basically agreed with me that the superposition of two atoms "technically" looked like an oscillating charge, but we were not allowed to use Maxwell's Laws to analyze it! When I pointed out that if you did treat them as little antennas, you get the correct values for things like the Einstein A and B coefficients, they said "even if that was true", it was just a coincidence. Yes, technically an atom may be in a superposition of two states, and the "probable" charge location may be oscillating, but there is no radiation associated with that oscillating "probability".

I'm going to try and say it clearly once and for all: everything that atoms do on a thermal level can be understood by following the motion of the charges and applying the classical Maxwell equations of electromagnetism. Schroedinger tried to argue this case for a couple of years but he was howled down by the "mean" physicists. There was actually an experiment done in 1929 which was considered decisive at the time, but which I am convinced has been misinterpreted. The problem with these arguments is that they are conducted on a very abstract level. Physicists, even those at the very top, are desperatley lacking in good physical pictures. That is why my explanation of the crystal radio is so very important. I am continually encountering people who should know better who make grevious mistakes in their explanation of the photoelectric effect because they simply do not have the picture in their heads, which I have in mine, of how a crystal radio absorbs power. I'm going to name one Marlon Scully in particular, a leading proponent of the Jaynes school which famously promoted the "semi-classical" interpretation of quantum optics. I think I'm going to save this discussion for my next blog post.

The Crystal Radio

It's hard to believe I've been posting for over a year and a half and I still haven't gotten around to talking about the case of the crystal radio, which was my personal epiphany on the road to quantum mechanics. I started toying around with the question when I was in fourth-year engineering: specifically, how much power could you absorb with a well-designed crystal radio set?

There was a time when everyone knew what a crystal radio was. There was no electricity out on the farms, and batteries were expensive. Ingenious people figured out that they could pick up radio stations with little more than an antenna, an earphone, a tuning coil and something called a "crystal".

The crystal is actually the least important part of the discussion which follows; all it does is rectify the radio-frequency power so it is audible in the earphone. From the perspective of energy flow, the power has already been absorbed before it is rectified. So we're going to ignore the crystal from here on in. The question is: given a fixed amount of power density from the distant radio transmitter, how much can you absorb with your small receiver station?

It seems obvious that the key to absorbing power lies in the design of the antenna; obviously, a bigger antenna will absorb more power than a smaller one. The problem is that the actual mechanism of power absorption by an antenna is somewhat obscure. People talk about an induced voltage, and in engineering class we did a lot of calculations on the voltage for different antenna configurations. But we never converted this into power. I knew there was something called the "radiation resistance" of an antenna, and that for maximum power transfer you wanted to match the radiation resistance to the resistance of the load (that would be the earphone); but none of this made much physical sense. How would you calculate this so-called "radiation resistance", and what was its physical meaning?

This went on for several years without much progress. Then I got in a discussion with some professors and one of them said that there was a simple formula for radiation resistance, and it went as the square of the antenna length. I had already given some thought over the years to the implications of various length dependencies, and had quickly ruled out a quadratic relationship because in combination with the well-known V-squared-over-R formula, it would imply that the theoretical absorbed power was independent of antenna length. I tried to make this point but the professor simply wandered off.

Still, something was fishy. I don't know how it came to me but I started drawing picturtes of radio waves: straight plane waves arriving from a distant stationon the left, and circular outgoing waves emanating from the receiving antenna as a result of the currents induced in it. Something like this:

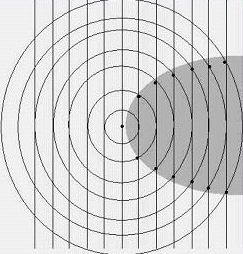
Notice that within the shaded region behind the antenna, the phase difference between the incoming and outgoing waves is everywhere less than 180 degrees; so within this zone, and only within this zone, there is the prospect of destructive interference. Why do I say the "prospect"? Because the nature of the interference will depend on the exact relative phase of the two wave patterns.

The amazing thing is we can do a rough calculation on the energy balance from this simple picture. Over most of the spherical surface of the outgoing wave, power is simply being re-radiated, or in other words, wasted. It is only in the shadow region that we can absorb the power that will ultimatley be delivered to the earphone. And here is the key: in the shadow region, the power removed from the incoming wave is in linear proportion to the induced current. In the remainder of the spherical area, the power re-radiated is proportional to the
square of the current.

Now, whenever you have two quantities, and one is linear and the other is quadratic, the linear one dominates at first; but then the quadratic grows faster, so it catches and overtakes the linear term. What this means for the antenna is that as you draw current and deliver it to the load, at first you have power available because the power you remove from the shadow zone is greater than the power you waste re-radiating into the rest of space. But as you try to draw more power from the antenna, the wasted power grows much faster than the useful power. The maximum current in the antenna flows when the load is short circuited; in that case, the power removed from the shadow zone is exactly equal to the power re-radiated to the rest of space. The maximum absorbed power is obtained at exactly one-half of this short-circuit current.

How do we contrive to get just the right amount of current to flow? This is called "matching the load impedance". And returning to the question we raised earlier: how do we arrange so that the relative phases of the two wave systems are just right so that there is maximum destructive interference in the shadow zone? This is called "tuning" the antenna, and it is the reason you have a tuning coil.

What does this have to do with quantum mechanics? The amazing thing in this calculation is that we have determined the amount of power we can absorb from an external wave, and nowhere in the calculation did we need to worry about the size of the receiving antenna! It doesn't matter how small you make the antenna: you can still absorb just as much power. Of course, there are practical limitiations; because of the electrical resistance of copper, you cannot easily demonstrate this effect with ordinary AM-radio receivers. However, the result is true in principle, and incredibly enough, nature provides us with abundant examples of perfectly ideal, lossless antenna systems. They are called "atoms" and they are everywhere.

What the crystal radio teaches us is that the absortive capacity of an atom at its resonant frequency is independent of the size of the atom. At least, this is what it should teach us if we are paying attention. But if we look at any high-school physics textbook, it tells us that classical physics cannot explain the photo-electric effect because the cross-sectional area of an atom is much too small to absorb the amount of energy needed to eject an electron from an atom. Hence the photon!

What the books don't say is that according to the same argument, a crystal radio shouldn't work. Because the antenna is made of a thin wire, and if you calculate the amount of energy actually falling on that wire, it is much to small to drive even the tiniest speaker. It's the same argument.

Does the crystal radio work? I will give the final word to my father. He grew up in the depression, and when I first got interested in this topic I asked him if he had ever heard of a crystal radio. "Oh, sure," he said. Did he get one to work? "I never saw one work. The Ukranian kids could make them work." (The North End of Winnipeg was characterized in those days by its ethnic population composed largely of Ukranians and Jews.)

It's funny that the Jews come in handy when you need to do something super technical like inventing the atom bomb or finding the cure for polio, but we don't seem to have the most practical hands-on skills in everything. I guess it goes to show that it takes all kinds.

TEN THINGS WE JEWS BELIEVE ABOUT THE MIDDLE EAST

I was pretty amazed to find out the other day that Google blogger tracks my hits, and that people have actually been reading my articles. I had no idea. I'm motivated to start writing a lot more stuff. But first I think I'm going to repost something I circulated by email in 2006, when there was still time for us to turn around and change our attitudes. A lot has happened in the last five years, and I'm afraid it's too late for me to do any good now, but I still want to have it on the record. It's my list of commonly held beliefs among the Jewish people that I believe are the real reason we won't settle with the Arabs. Here they are:

1. The Oslo process was doomed to failure.

Who wrecked Oslo, us or them? The "process" lasted exactly eight years, from the initial signing until the outbreak of the intifada. In all that time we gave them thirteen percent of the West Bank and doubled the settler population from 100,000 to 200,000. How did we expect them to respond?

2. Force is the only language they understand.
And are we any better? During the intifada, I applauded our targeted killings and other military countermeasures. But when the Palestinians managed to hold their fire for five or six weeks at the height of the violence, did we respond withthe positive gestures (e.g. prisoner releases) that would have given the moderatessomething to show for their efforts? No we did not. So they went back to bombingus, because that is the only thing that gets our attention.

3. Hamas and Hizbullah are terrorists, and we don't negotiate with terrorists.
Are they really now? Read a list of their operations. Mostly they attack Israeli military targets. Shelling cities? Not very nice of them, but is that terrorism? The allies bombed Germany and Japan in WWII, and we don't call that terrorism. Classic terrorism is all about hijacking airplanes, shooting up schoolhouses, and blowing up cafes. Not exactly Hizbullah's style.

4. We made the desert bloom.
Well good for us, but so what?

5. The Palestinians already have a state, and it is called Jordan.
There is actually a grain of truth to this claim, but once again: so what? The Jews also have a state, and it is called Florida.

6. There is no one to talk to.
Wrong. This might have been the case immediately after the "Three No's" (Khartoum 1967), but it hasn't been true for years. Nowadays we are the ones who refuse to talk. And when positive overtures come forward from the other side, we just scoff at them. (Arab League peace plan, Beirut 2002?)

7. The "Right of Return" is a plot to destroy Israel demographically.
The Arabs are not stupid. Every Arab who talks about peace knows that the unrestricted "Right of Return" is a deal-breaker for us.

8. The two sides will never be able to agree on how to divide Jerusalem.
Have you been to Jerusalem recently? I have. It's already divided.

9. The Arabs are just liars anyways.
This is just such racist crap that I'm not even going to respond to it.

10. ***WE ARE BETTER THAN THEM***
More racism, but so fundamentally ingrained that it MUST be dealt with. Listen: we Jews are a clever people and we have a right to be proud of our accomplishments. But all the Nobel prizes in the world don't make us BETTER than the Arabs. We are all just PEOPLE. Get over the "chosen" bullshit.

=======================

My solution to the conflict:

1. Israel accepts the Saudi proposal and recognizes an independent Palestine
within the 1967 borders.

2. Palestine accepts the Barak proposal and gives Israel a 99-year lease on
three percent of the West Bank.

The Collapse of the Wave Function

I didn't know when I started this blog two years ago that within a few months I would figure out how to explain one of the most baffling phenomena of quantum mechanics: the collapse of the wave function. I've already written it up under the title of "Quantum Siphoning" but in that article I dealt in detail with the actual mechanism. Today I'm going to revisit the "big picture".

I've taken as the prototypical example of wave function collapse to be the appearance of flecks of silver on a photographic plate when exposed to the weak light of a distant star. The reduction of silver bromide is a chemical process that requires a significant input of energy. You do not get a silver fleck on the plate without the reduction of silver bromide. So where does the energy come from? Obviously, it must come from the light wave. But it is a fact that we can make the light source weaker and weaker without limit, and yet all we have to do in order to see flecks appear is to wait long enough. How can we explain this unless we accept that the energy of the light, contrary to Maxwell's Laws, is present in concentrated lumps?

The answer to this paradox is suttle and unexpected. We must consider the reduction of silver bromide from a thermodynamic perspective. In thermodynamics we do not talk about A and B reacting to form C; we say that A, B, and C are in equilibrium; and that equilibrium occurs when the rate of combination of A and B into C is equal to the rate of decomposition of C into A and B. Everything is in flux.

We are taught to calculate the point of equilibrium by means of the Gibbs Free Energy Function. How does this apply to a photographic film? Here we must consider the equilibrium between silver bromide and metallic silver. The Gibbs Free Energy function tells us that it takes a great amount of energy to convert silver bromide to metallic silver. But that is only part of the story. The Gibbs Free Energy is calculated for reactants and products in their stoichiometric proportions: in this case, equal molar fractions of silver bromide and metallic silver. In fact, this is far from the case in an unexposed photographic plate.

In an unexposed film there is virtually no metallic silver; it takes only a few parts per billion of silver in a single crystal to render a developable image. To calculate the thermodynamics, we must then consider the crystal as a solid solution of reactants and products; and when the Gibbs Free Energy is recalculated taking this into account, it turns out that the equilibrium point is indeed on the order of parts per billion.

What does this mean? In equilibrium, the forward and backward conversion rates are equal. Silver is turning into silver bromide, and silver bromide is turning back into silver of its own accord. There is no net input of energy required to drive either the forward or reverse process. When a silver bromide atom turns to silver, or vice versa, there is no net change in the energy of the entire crystal.

If this is the case, then there is no valid argument whereby the energy of light must be delivered in concentrated lumps in order to drive the reaction. The conversion may be readily catalyzed by the smallest amount of light energy, because the energy needed for the transition is already present in the crystal.

I have worked out the mathematics of this and posted my calculation in this discussion on physicsforums.com. I'm going to repost it on my blog later, but for now you can check it out as post 44 in the discussion. The funny thing was that I wasn't really prepared to make this point when it came up. I had originally been arguing that the reduction of silver was spontaneous in the "ordinary" sense of having a negative free energy by the simple (stoichiometric) calculation, and I was shocked to find that I was wrong about that.

The reason I was able to recover was that twenty-five years earlier, as a young engineer, I had been exposed to a peculiar situation where a hydrocarbon detector was giving odd results. It was not showing the expected presence of methane in CO2, and I put forward the theory that the methane and CO2 were reacting to form carbon monoxide. I was ridiculed for this because the Gibbs Free Energy of my reaction was obviously positive, so everyone assumed it couldn't move forward. What they ignored was the effect of concentration. I was able to show that at the trace concentrations we were looking at (parts per million) the point of equilibrium actually tipped in the opposite direction. (There was also an elevated temperature to consider.) I've described this in a series of posts from earlier this year.

The point is that its the exact same thermodynamic calculation that turns the traditional explanation of "wave function collapse" on its head. The notion of collapse is based on the assumption that the energy for the process must have come from the photon, and I am able to show that the energy is already present in the detection system (the photographic plate.) If it's true in this prototypical instance of wave function collapse, then how many other situations might also be analyzed in this way?